Explicit Formula For Geometric Sequences

An explicit formula is used to calculate the nth term of a sequence by explicitly or direct putting in the value of northward .

For instance, if you want to determine the $half-dozen^{th}$ term of the sequence, and then you volition put $northward = 6$. The explicit formula is generally written as $a_{n} = a + (north-1) d$, but this formula is used to determine the terms of an arithmetics sequence. We can utilise the explicit formula to find the terms of arithmetics, geometric and harmonic sequence.

In this article, we volition talk over in detail different sequences and their explicit formulae, forth with numerical examples.

What Is an Explicit Formula?

An explicit formula is a formula that is used to determine the $n^{th}$ term of different types of sequences.

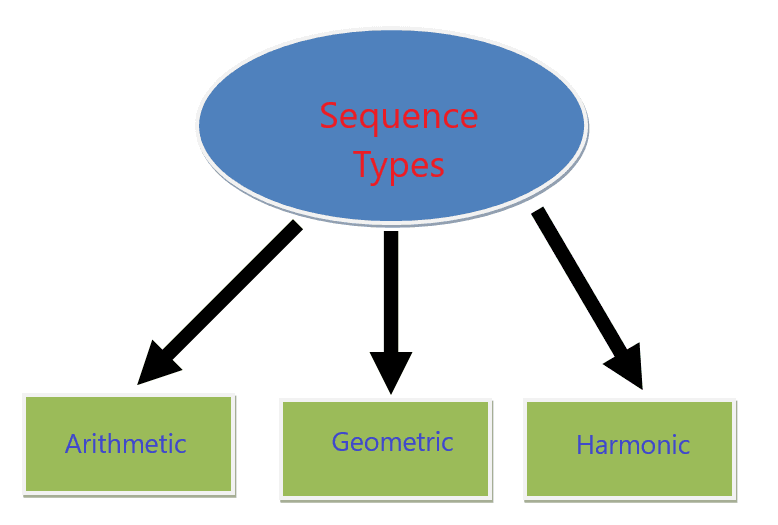
There are different types of explicit formulae, mainly divided into three types, i.east., arithmetics, geometric and harmonic sequences. Explicit means direct or exact; hence, when applied correctly, we can calculate any term of the given sequence immediately.

What Is a Sequence?

A sequence is a serial of numbers which share a common blueprint. The sequence can be finite or infinite. The infinite sequence has three dots at the finish. For instance, $1$,$ii$,$3$,$four$… volition exist called an infinite sequence, while $1$,$2$,$3$ will be called a finite sequence.

The numbers in the sequence are called terms. For example, in the sequence, $1$,$two$,$iii$, the number "$1$" is called the 1st term of the sequence and similarly, the number $3$ is chosen the $3rd$ term of the sequence. There are different types of sequences, merely for this topic, we will talk over arithmetic, geometric and harmonic sequences.

Arithmetics Sequence

An arithmetic sequence is a sequence in which the common deviation between the terms of the sequence remains constant. We can also define an arithmetics sequence as a sequence in which the same number is added or subtracted to each term of the sequence to generate a constant pattern.

In the sequence $0$,$2$,$iv$,$6$, $8$, nosotros are calculation "two" to each term of the sequence, or we can say that the mutual divergence is "$two$" between each term of the sequence.

Geometric Sequence

A geometric sequence is a type of sequence in which each term is multiplied by a constant number, or we tin can also ascertain it as a sequence in which the ratio of the consecutive terms or numbers in the sequence remains abiding.

For example, suppose nosotros were given a sequence of $two$,$4$,$8$,$16$,$32$ and so on. In this sequence, we multiply each term by the number "$2$". Note that the ratio between consecutive terms remains the same. The ratio between $iv$ and $2$ is $\dfrac{4}{2} = 2$; similarly, the ratio between $8$ and $iv$ is $\dfrac{8}{4} = two$.

Harmonic Sequence

A harmonic sequence is a type of sequence which is inverse of the arithmetic sequence. For case, if we are given an arithmetics sequence of $x_{1}$,$x_{2}$,$x_{three}$… then the harmonic sequence volition exist $\dfrac{1}{x_1}$, $\dfrac{1}{x_2}$,$\dfrac{one}{x_3}$. The harmonic sequence or harmonic progression is merely the reciprocal of an arithmetic sequence.

Explicit Formula for an Arithmetic Sequence

We tin can employ the explicit formula for an arithmetics sequence to determine any term of the sequence, fifty-fifty if express data is provided for the sequence. As the name explicit means direct, nosotros can directly detect out a specific term without computing the terms before and after it.

Suppose nosotros want to decide the 8th term of the sequence, and so it is not necessary to notice out the $vii^{thursday}$ or $9^{th}$ terms before calculating the $eight^{th}$ term of the sequence.

The explicit formula for an arithmetics sequence is given as

$a_n = a + (n-1) d$

Hither:

a = Showtime Term of the sequence

d = common difference

n = number of the term

Allow us report an example related to the arithmetic sequence. For example, nosotros are given a sequence $ane$, $five$, $9$, $13$, $17 \cdots$. The first term of the sequence is $ane$, hence $a = 1$. We can calculate the mutual deviation past subtracting two consecutive terms $d = five – 1 = 4$ or $d = 9 – 5 = 4$. Now that we have the value of the get-go term and the common difference of the sequence, we can find the value of any term of the sequence. Say we want to find the value of the $10^{th}$ term of the sequence, and then $n = ten$.

$a_{10} = i + (x – one) 4$

$a_{10} = i + (9) 4$

$a_{10} = 1 + 36 = 37$

So the $10^{th}$ term of the sequence is $37$.

Permit us study some explicit formula examples.

Example 1: Determine the first three terms for the given arithmetic sequences.

$a = 3$ and randomly chosen 3 consecutive terms are $39$,$42$ and $45$
$a = i$ and randomly called three sequent terms are $36$,$43$ and $50$
$a = nine$ and randomly chosen three consecutive terms are $54$,$59$ and $64$

Solution:

1).

We have to calculate the offset three terms of the arithmetic sequence.

First, second, and third term tin can be calculated equally $n = 1$ , $n = 2$ and $n = iii$ respectively.

The common difference for this sequence is $d = 42 – 39 = iii$.

$a_{i} = iii + (1 – i) 3 = iii$, $a_1 = a = 3$

$a_{two} = 3 + (2 – 1) iii = 3 + 3 = 6$

$a_{3} = 3 + (3 – ane) 3 = 3 + 6 = nine$

ii).

The common divergence for this sequence is $d = 43 – 36 = vii$.

$a_{1} = one + (1 – one) 7 = 1, a_1 = a = 1$

$a_{two} = 1 + (2 – 1) 7 = 1 + seven = viii$

$a_{3} = ane + (3 – ane) 7 = 3 + 14 = fifteen$

3).

The mutual difference for this sequence is $d = 59 – 54 = 5$.

$a_{ane} = 9 + (1 – 1) five = nine$, $a_1 = a = 9$

$a_{ii} = ix + (2 – 1) 5 = 9 + five = 14$

$a_{iii} = 9 + (3 – 1) v = ix + 10 = 19$

Example 2: Calculate $n$ for an arithmetic sequence having $a = 10$, $a_{north} = xc$ and $d =10$.

Solution:

We know the explicit formula for an arithmetics sequence is given every bit:

$a_{n} = a + (n-1) d$

$xc = x + (n -1) 10$

$eighty = (n-1) 10$

$viii = n – 1$

$n = 9$

Explicit Formula for Geometric Sequence

We can employ the explicit formula for the geometric sequence to observe out whatsoever term of the geometric sequence. For the explicit formula of the arithmetics sequence, we crave the showtime term and the common difference to notice out the $n^{th}$ term of the sequence. In this example, we need the first term and the common ratio.

The common ratio of the geometric sequence tin can exist calculated by taking the ratio of the ii consecutive numbers in the sequence. A generic geometric sequence is given equally $a$, $ar$, $ar^{2}$, $ar^{3}$, $ar^{4}$… $ar^{n-1}$. The explicit formula for the geometric sequence is given as:

$a_{northward} = ar^{n-1}$

Hither:

a = First term of the sequence

r = common ration = $\dfrac{ar}{a}$ or $\dfrac{ar^{2}}{ar}$

Say we are given a geometric sequence $one$,$6$,$36$, $216$… and we demand to discover out the $7^{thursday}$ term of the geometric sequence. Here, $a = 1$ while $r = \dfrac{6}{1}= 6$ or $r = \dfrac{36}{6} = 6$. We want to detect the 7th term using the explicit geometric sequence formula.

$a_{7} = 1 \times (vi)^{7 – 1} = ane \times vi^{6} = 46,656$

Example iii: Determine the fifth and sixth terms for the given geometric sequences.

1. $4$,$viii$,$12$,…

2. $seven$, $14$, $21$, $28$…

Solution:

ane).

We are given the start three terms of the sequence. So $a_{1} = iv$, $a_{2} = 8$ and $a_{3} = 12$

Mutual Ratio $= r =\dfrac{a_2}{a_1}= \dfrac{viii}{four} = ii$

We need to discover the 5th and sixth terms of the sequence, and we know the explicit formula for the geometric sequence is:

$a_{n} = ar^{n-i}$

$a_{5} = 4.(two)^{five-ane}$

$a_{five} = 4.(ii)^{4} = 4 \times 16 = 64$

$a_{six} = iv.(2)^{six-1}$

$a_{6} = 4.(2)^{5} = iv \times 32 = 128$

2).

Nosotros are given the showtime 4 terms of the sequence. So $a_{ane} = 7$, $a_{2} = xiv$, $a_{three}= 21$ and $a_{4} = 28$.

Mutual Ratio $= r =\dfrac{a_2}{a_1}= \dfrac{xiv}{vii} = 2$.

$a_{n} = ar^{n-one}$

$a_{5} = 7.(ii)^{5-1}$

$a_{v} = vii.(2)^{iv} = vii \times 16 = 112$

$a_{6} = seven.(ii)^{6-1}$

$a_{vi} = 7.(2)^{5} = vii \times 32 = 224$

Explicit Formula for Harmonic Sequence

We tin use the explicit formula for a harmonic sequence to determine whatever term in a given harmonic sequence. We know that a harmonic sequence is an inverse or reciprocal of an arithmetic sequence. The general representation of a harmonic sequence can be given as $\dfrac{i}{a}$, $\dfrac{one}{a + d}$, $\dfrac{i}{a+2d}$,…, $\dfrac{1}{a + (n-1) d}$. The explicit formula for the harmonic sequence is written as:

$a_{due north} = \dfrac{1}{a + (n-1) d}$

a = First Term of the sequence

d = common departure

n = number of the term

We can hands determine the value of any term of a geometric sequence using the above-mentioned explicit formula. Say nosotros are given a harmonic sequence $\dfrac{1}{3}$, $\dfrac{ane}{6}$, $\dfrac{1}{9}$,$\dfrac{1}{12}$… Let usa first consider if the arithmetics sequence is corresponding to this harmonic sequence. The first term of that arithmetics sequence is $a = 3$ while the mutual difference $d = 6 – 3 = 3$ or $d = 12 – 9 = 3$. Suppose we need to detect the 9th term of the harmonic sequence. Applying the explicit formula:

$a_{9} = \dfrac{i}{3 + (9-ane) 3}$

$a_{nine} = \dfrac{1}{3 + (8) iii} = \dfrac{1}{3 + 24} = \dfrac{1}{27}$

Example 4: If the $five^{th}$ and $8^{th}$ terms of a harmonic sequence are $\dfrac{3}{seven}$ and $\dfrac{3}{13}$, respectively, find out the harmonic sequence by using these terms.

Solution:

We tin say that the $v^{thursday}$ and $8^{thursday}$ terms for the arithmetic sequence, in this case, would exist $\dfrac{8}{3}$ and $\dfrac{14}{iii}$, respectively. And then:

$a_{5} = a + 4d = \dfrac{seven}{3}$ (1)

$a_{8} = a + 7d = \dfrac{xiii}{3}$ (2)

Subtracting equation (ane) from (2), nosotros will get:

$3d = \dfrac{13}{three} – \dfrac{seven}{three} = \dfrac{6}{3} = two$

$d = \dfrac{2}{iii}$

Putting the value of the common difference "d" in equation (1):

$a + iv (\dfrac{2}{3}) = \dfrac{seven}{three} = \dfrac{7}{3} – \dfrac{8}{3} = -\dfrac{1}{3}$

So, $a = a_{1} = -\dfrac{1}{three}$

Remember this $a_{1}$ is for the arithmetic sequence.

Let us now calculate the second, third and fourth term.

$a_{2} = a_{1} + d = -\dfrac{1}{iii} + \dfrac{two}{3} = \dfrac{1}{3}$

$a_{three} = a_{1} + 2d = -\dfrac{ane}{3} + two (\dfrac{2}{three}) = 1$

$a_{4} = a_1 + 3d = -\dfrac{ane}{iii} + 3 (\dfrac{2}{three}) = \dfrac{v}{iii}$

Now, if we take the reciprocal of the above terms, then we will get the harmonic sequence or progression:

$\dfrac{3}{(-i)}$, $\dfrac{3}{(one)}$, $1$, $\dfrac{3}{5}$, $\dfrac{3}{7}$,…

Steps to Apply the Explicit Formulas

If we are dealing with an arithmetic sequence, and then we know the formula for the $due north^{th}$ term is $a_{n} = a + (n-one)$ d, and then all we need to exercise is to find the value of "$a$" and "$d$", and we will take the final equation for the $n^{th}$ term of the arithmetic equation. The $n^{th}$ term for an arithmetic sequence can be evaluated using the explicit formula using the steps given below.

The first footstep is to find the common difference and the starting time term of the sequence.
Put the values of the commencement term and common divergence in the $n^{th}$ term formula.
Solve the equation to get the $n^{th}$ term formula for the arithmetics sequence.

The explicit formulae for geometric and harmonic sequences can besides be practical using the same method. For geometric sequence, you need to find out common ratio instead of common difference, while for harmonic sequence, but follow the procedure of arithmetic sequence and take the changed at the end.

Example 5: If $a_{due north-3} = 4n – 11$, so what will exist the $northward^{th}$ term of the sequence?

Solution:

We are given an explicit formula for the sequence, and with the help of it, we need to determine the sequence'due south $northward^{th}$ term. Showtime, nosotros need to discover out $a_{1}$ and $d$. Let u.s.a. observe out the first three terms of the sequence at n = $4$,$v$,$vi$.

$a_{4-3} = 4(4) – 11 = a_1 = 16 -11 = five$

$a_{5-3} = v(4) – 11 = a_2 = 20 -11 = 9$

$a_{6-three} = 6(iv) – xi = a_3 = 24 -eleven = 13$

So the get-go iii terms of the sequence are $5$,$9$,$13$.

The mutual difference of the sequence $d = ix – 5 = 4$.

$a_{n} = 5 + (northward-1) four$

$a_{n} = 5 + 4n- iv$

$a_{n} = 4n + 1$

Example six: Determine the $n^{th}$ term of the geometric sequence if $\dfrac{a_7}{a_5} = \dfrac{16}{nine}$ and $a_{2} = \dfrac{4}{ix}$.

Solution:

Nosotros can write $a_{seven} = a_1.r^{half dozen}$ and $a_{v} = a_1.r^{iv}$.

$\dfrac{a_7}{a_5} = \dfrac{sixteen}{ix}$

$\dfrac{ a_1.r^{6}}{ a_1.r^{four}} = \dfrac{xvi}{ix}$

$r^{2} = \dfrac{16}{9} = \pm \dfrac{4}{iii}$

We know that $a_{2} = a_{1}.r$

$a_{2} = \dfrac{4}{9}$

$a_{1}.r = \dfrac{four}{9} = a_{ane} = \dfrac{four}{9r}$

So, when $r = \dfrac{4}{three}$ so $a_{1}$ will exist

$a_{1} = \dfrac{4}{ix.\dfrac{4}{3}} = \dfrac{4}{12} = \dfrac{1}{3}$

Then when $r = -\dfrac{4}{three}$, then $a_{1}$ will be:

$a_{1} = \dfrac{four}{9.(-\frac{4}{three})} = -\dfrac{iv}{12} = -\dfrac{1}{three}$

So when $r = \dfrac{4}{three}$ and $a_{1} = \dfrac{1}{3}$, then the $n^{th}$ term of the sequence volition exist:

$a_{n} = ar^{northward-i}$

$a_{n} = \dfrac{i}{three}.(\dfrac{4}{3}) ^{due north-one}$

When $r = -\dfrac{4}{3}$ and $a_{1} = -\dfrac{1}{3}$, then the $n^{thursday}$ term of the sequence volition be:

$a_{n} = ar^{due north-1}$

$a_{n} = -\dfrac{1}{iii}.(-\dfrac{4}{3}) ^{northward-ane}$

Example 7: Make up one's mind the $7^{th}$ and $due north^{th}$ term of the harmonic sequence $\dfrac{1}{3}$,$\dfrac{1}{5}$,$\dfrac{one}{7}$,…

Solution:

If we take the reciprocal of the sequence, it will requite us the arithmetics sequence. We can write the arithmetic sequence as $3$,$five$,$seven$…

Here $a = 5$ and $d = five-3 = two$

$a_{due north} = a + (northward-1) d$

$a_{northward} = 5 + (n -1) two$

$a_{n} = 5+ 2n -2 = 2n + 3$

And so the $northward^{th}$ term of the harmonic sequence will exist:

$\dfrac{1}{ a_{n} } = \dfrac{1}{2n + iii}$

We can easily calculate the 7^{th} term of the sequence now past putting $n = vii$.

$\dfrac{1}{ a_{seven}} = \dfrac{i}{2(vii) + 3} = \dfrac{ane}{17}$

Example 8: Suppose a theatre has $ten$ rows, and the seats from row $1$ to row $10$ follow a specific pattern. The total number of seats in the first row is $6$ while the number of seats in the 2d is $eight$ and in the third-row, the full number of seats is $ten$. By using the explicit formula, make up one's mind the number of seats in the $9^{th}$ row.

Solution:

We can write the sequence as $vi$,$8$,$10$,…

And so here, $a_{1} = half-dozen$ and $d = viii-6 = 2$ and as we want to determine the number of seats in the $9^{thursday}$ row, hence $n = ix$. The explicit formula is:

$a_{n} = a_1 + (northward-one) d$

$a_{9} = 6 + (nine-1) 2 = 6 + 16 = 22$

So the number of seats in the $9^{thursday}$ row will be $22$.

Practise Questions

Observe out the explicit formula for the arithmetic sequences $iv$,$7$,$x$,$13$,$sixteen$…
Detect out the sixth term of the geometric sequence $v$,$15$,$45$,…
If the $vi^{thursday}$ term of the arithmetics progression is $14$ and the $20^{th}$ term is 42, what will be the value of $a_{due north}$ and $a_{thirteen}$?
What is a recursive arithmetics formula?
Determine if the sequence is arithmetics. If it is, notice the common difference and the explicit formula. 6,eight,nine,xi…

Reply Cardinal:

1).

$a = 4$

$d = 7 – 4 = 3$

$a_{n} = 4 + (n-i) 3 = 3n + ane$

two).

$a = five$

$r = \dfrac{fifteen}{five} = 3$

$a_{north} = a.r^{n-1}$

$a_{half-dozen} = 5. (three)^{6-one} = five \times 243 = 1215$

3).

$a_{6} = xiv$

$a_{20} = 42$

$a_{6} = a + 5d = 14 (one)$

$a_{20} = a + 19d = 42 (2)$

Subtracting eq (i) from (2):

$xiv d = 28$

$d = 2$

Putting the value of "d" in eq (1):

$a + 5 (2) = 14$

$a + 10 = 14$

$a = 4$

So now that we have the value of the first term and common departure "$d$", we tin easily find out the $n^{th}$ term of the sequence.

$a_{due north} = 4 + (north-1) ii = 2 (n +1)$

We can calculate the $xiii^{th}$ term by simply putting $n = 13$ in the to a higher place equation.

$a_{13} = 2 (13+i) = 28$

4).

Recursive and explicit formulas are not much different. Basically, Recursive formulas are drawn from explicit formulas. We know that the explicit formula for an arithmetic sequence is:

$a_{due north} = a +(n-1)d$

If we want to detect out the third term, we volition write $a_{iii} = a + (3-1) d = a_{1} +2d$ and we know that $a_{2} = a_{1} + d$, then nosotros can write $a_{iii} = a_{2} + d$. We can write the recursive formula for an arithmetic sequence as:

$a_{northward} = a_{n-1} + d$

v).

The sequence is not an arithmetic sequence because the common departure does not remain the same.

$d = 8 – 6 = 2$

$d = ix – 8 = 1$